This paper is available on arxiv under CC 4.0 license.
Authors:
(1) Nicolas Bernal, New York University Abu Dhabi;
(2) Partha Konar, Physical Research Laboratory;
(3) Sudipta Show, Physical Research Laboratory.
Table of Links
- Abstract and Intro
- S-matrix: Unitarity and its Consequences
- Dark Matter Annihilation and Unitarity Bound
- Low-temperature Reheating
- Freeze-out with a Low-temperature Reheating
- Summary and Conclusion
- Acknowledgments and References
5. Freeze-out with a Low-temperature Reheating
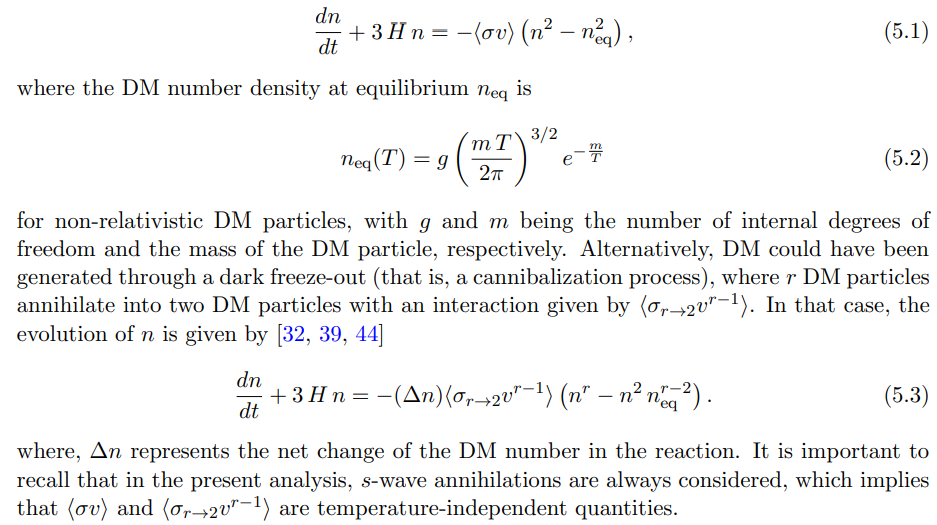
In this section, two cases for the DM freeze-out are considered. The first corresponds to the visible freeze-out, where a couple of DM particles annihilate into a couple of SM states, with a total thermally-averaged annihilation cross section ⟨σv⟩. The evolution of the DM number density n can be described with the Boltzmann equation [20]
5.1. Kination-like
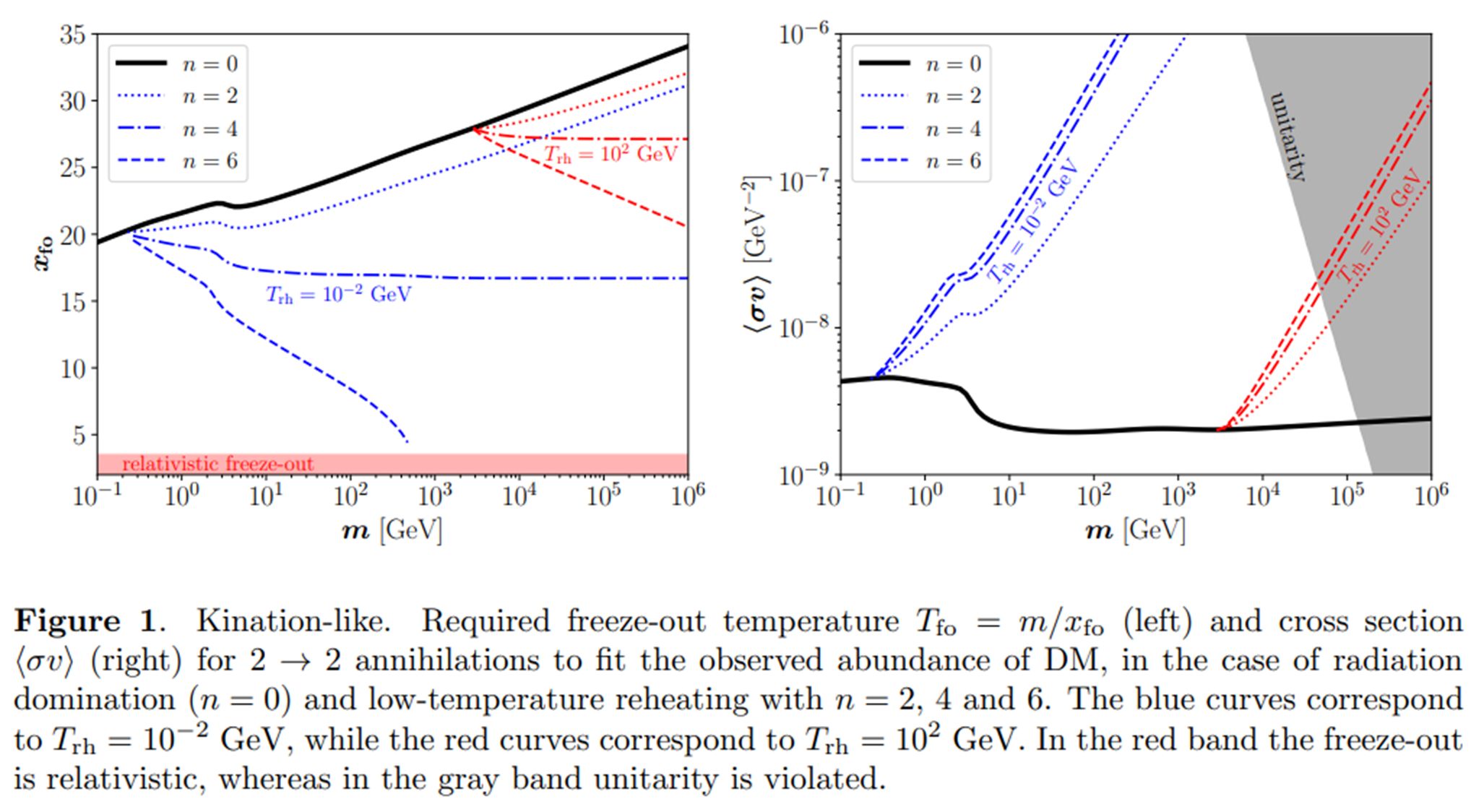
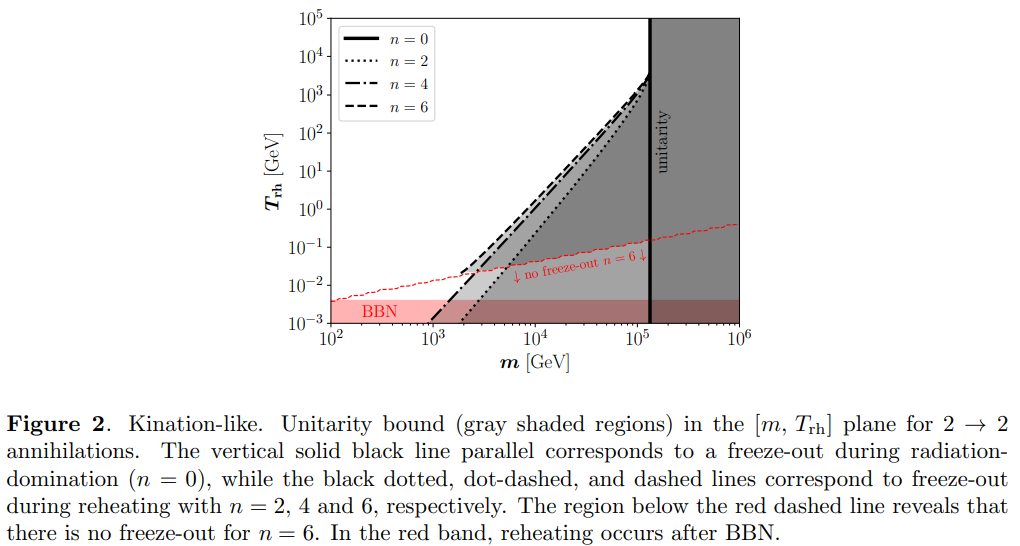
In the following, Eqs. (5.6) and (5.7) will be analytically solved in the context of a kination-like cosmology. For convenience, we start with the case corresponding to the dark freeze-out.
5.1.1. Dark freeze-out
If the freeze-out occurs during the radiation-dominated era, Eq. (5.7) can be analytically solved, from the DM freeze-out until today (i.e., small temperature and therefore large x)
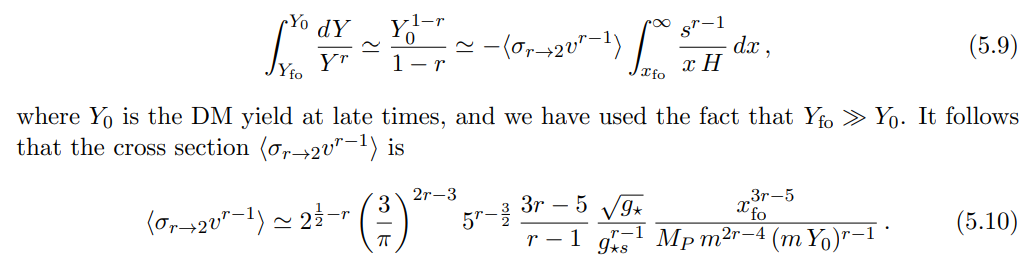
To match the whole observed DM relic density, it is required that
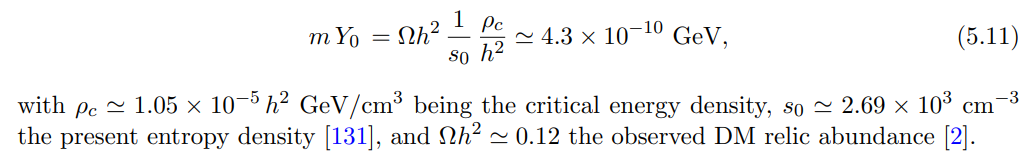
Alternatively, if the freeze-out happens during reheating
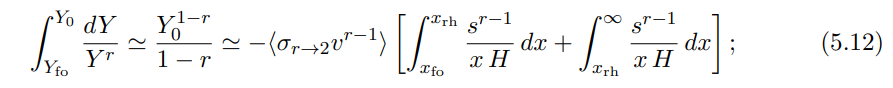
the integral has been split into two pieces, to emphasize the two regimes of H in Eq. (4.6). Therefore
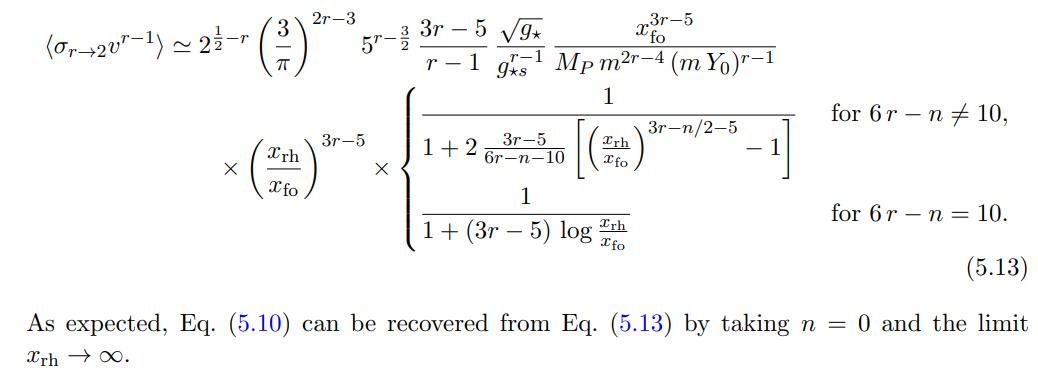
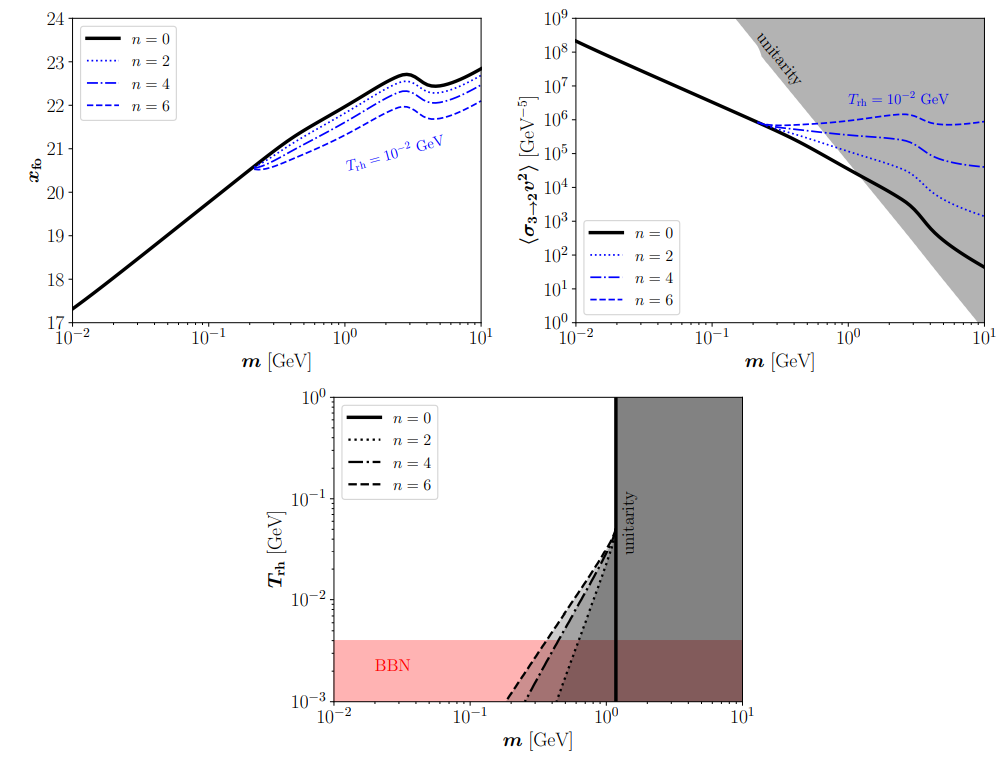
5.1.2. Visible freeze-out
The case of the visible freeze-out in Eq. (5.6) can be computed following the same procedure presented in the previous subsection. However, one could also derive it by fixing r = 2 in Eqs. (5.10) and (5.13), which gives
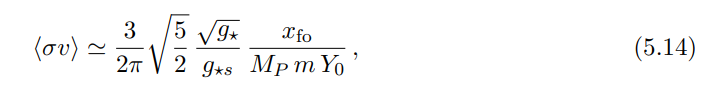
for the freeze-out in the radiation-dominated era, or
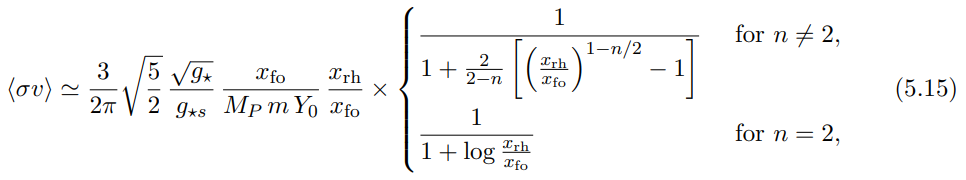
during reheating.
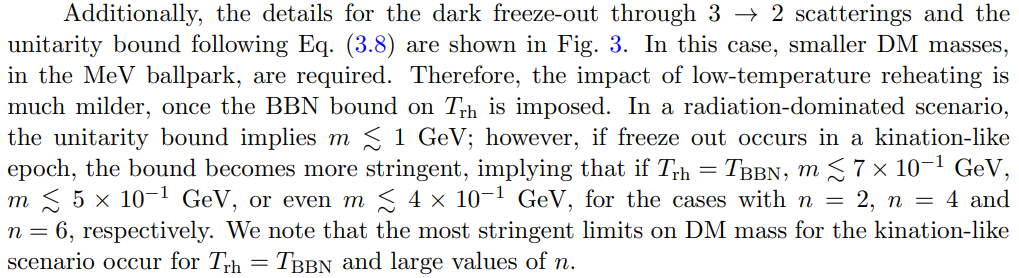
5.2. Early matter domination
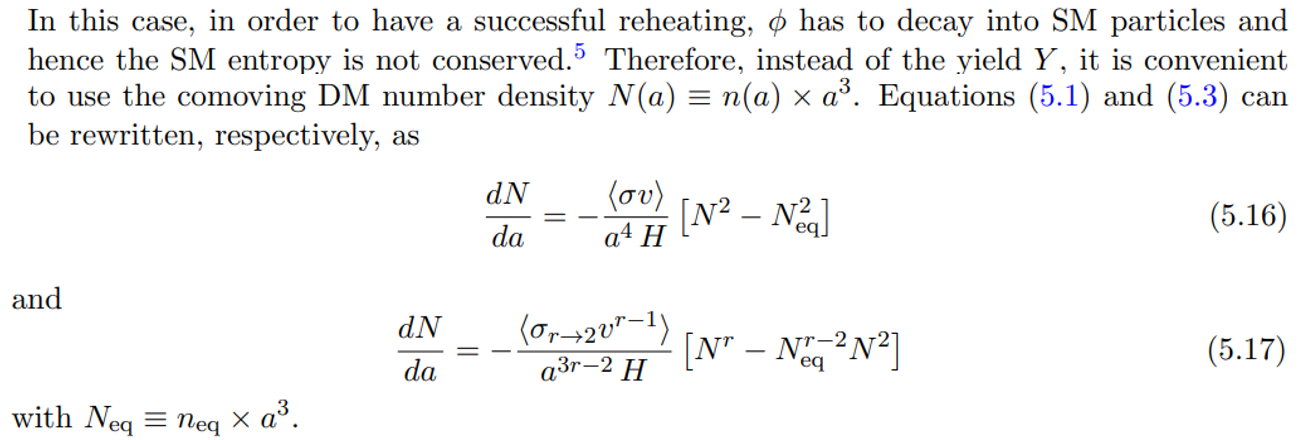
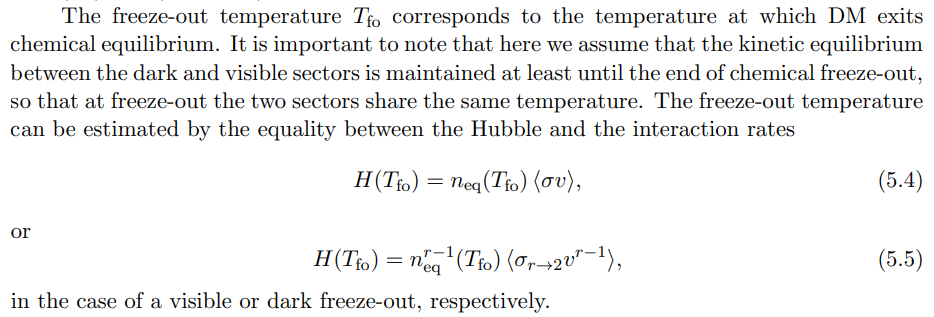
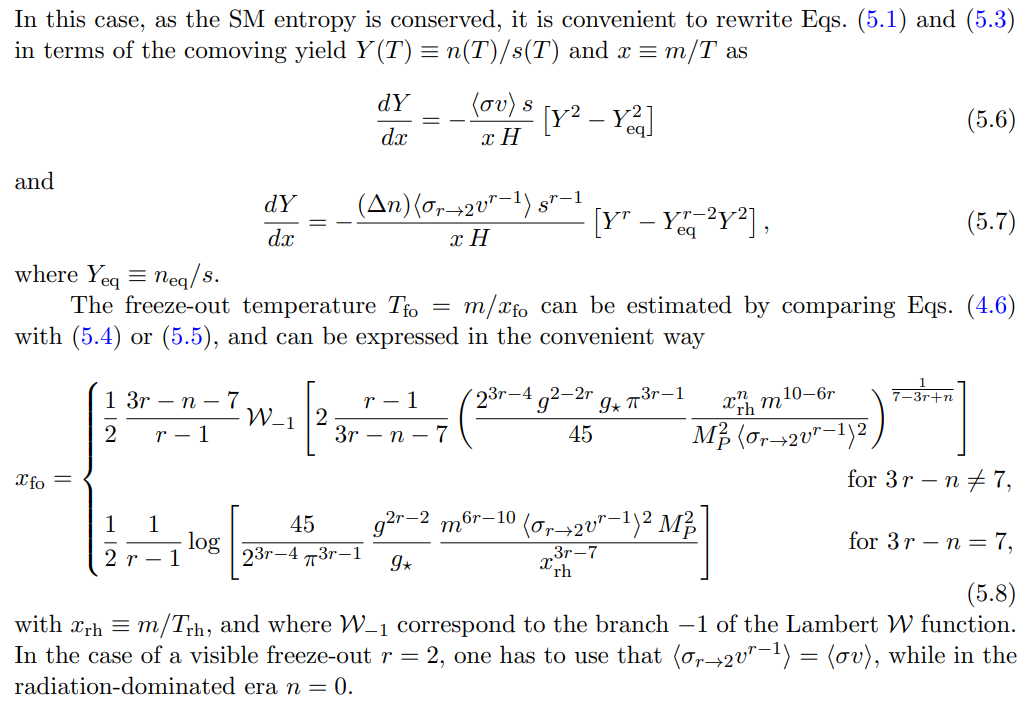
The freeze-out temperature can be estimated by comparing Eqs. (4.8) and (5.4) or (5.5), and is given by
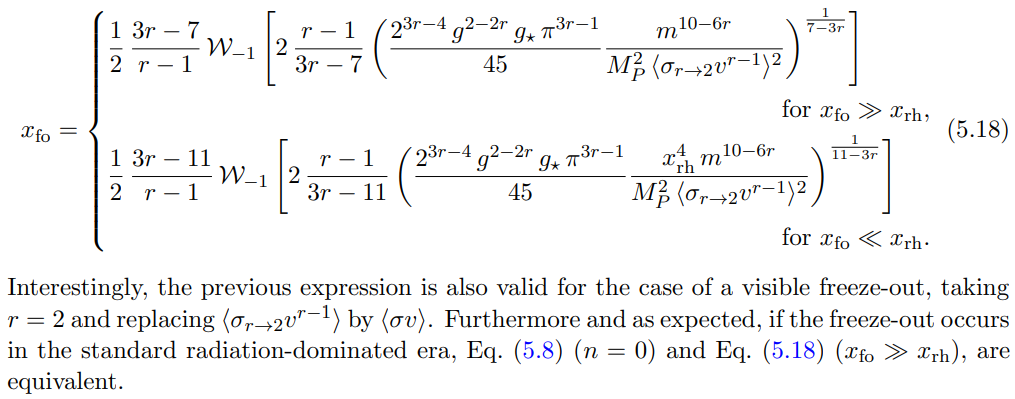
Next, analytical solutions are presented for Eqs. (5.16) and (5.17) in the context of an early matter-dominated scenario. We will begin with the case corresponding to the dark freeze-out for convenience.
5.2.1. Dark freeze-out
If the freeze-out occurs during the radiation era, the solution of Eq. (5.17), or equivalently of Eq. (5.7), is the one presented in Eq. (5.10). Instead, if it occurs during the reheating period, one has that
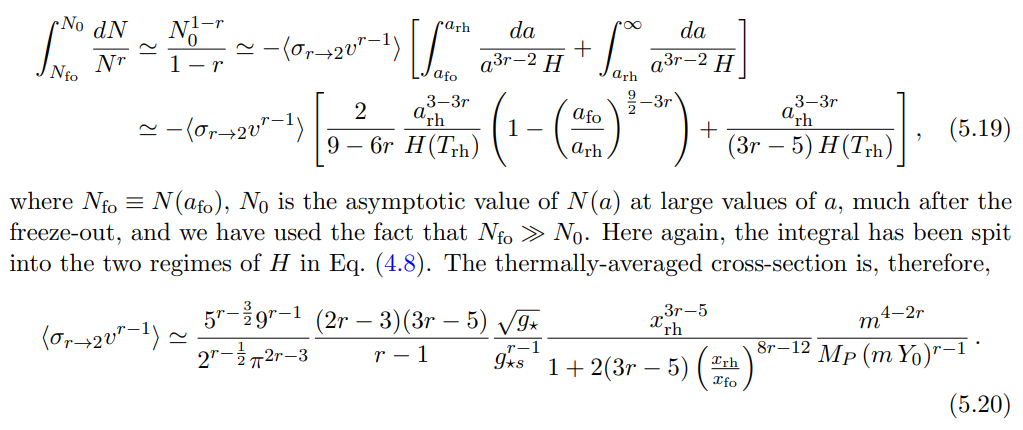
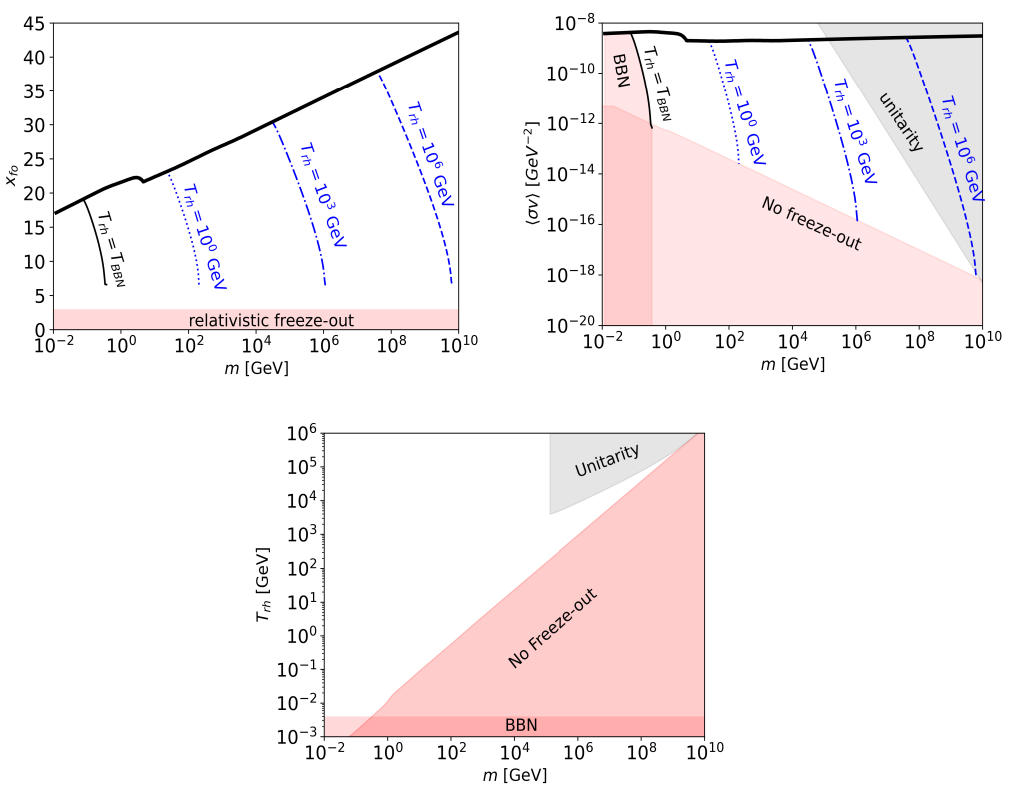
5.2.2. Visible freeze-out
If the freeze-out occurs during radiation domination, the solution of Eq. (5.16) is the same as the one of Eq. (5.14). Alternatively, if it occurs during reheating, one has instead
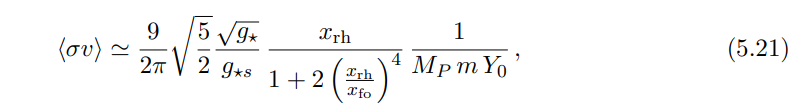
simply corresponding to the limit r = 2 of Eq. (5.20).